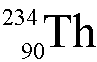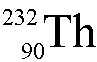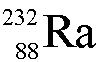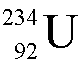Summary
- de Broglie hypothesis
- The Bohr model
- (The quantum mechanical model)
- lasers
- Written quiz Ch. 30-31
Chapter 32
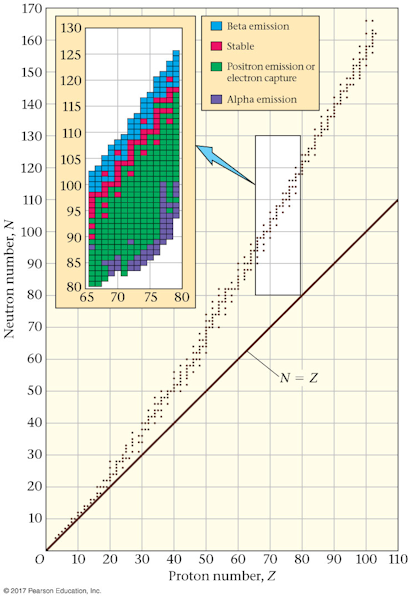
- The nucleus
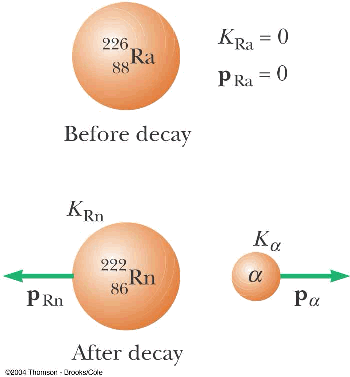
- Radioactive decay
- alpha decay
- beta minus decay
- gamma decay
Example #2
- Δm and the energy released
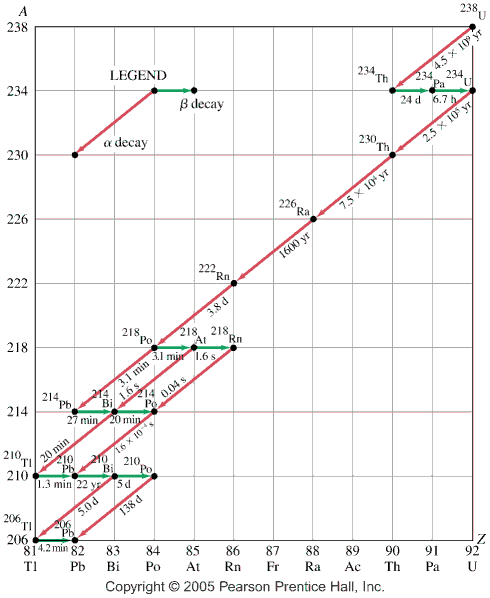
- Lecture learning outcomes
A student who masters the topics in this lecture will be able to:
- describe the constituents of the atomic nucleus and explain the meaning of isotope
- write balanced nuclear decay reactions for alpha, beta minus, or gamma decay, and identify either the parent or daughter isotope
- calculate the energy released in a nuclear decay reaction by determining the mass difference Δm in atomic mass units and multiplying the result by 931.5 MeV/c², a constant that is connected to Einstein's famous relationship, E = mc².
Practice:
Try these additional examples
Example #3
Example #4
Prepare:
Read textbook section 32-3 before the next lecture
gc6 30.q2
What isotope is represented by the X in
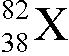 ?
?
A. xenon-82
B. lead-38
C. strontium-38
D. strontium-82
Answer
gc6 30.23mod
Uranium-236 (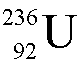 ) undergoes alpha decay.
What is the daughter nucleus?
) undergoes alpha decay.
What is the daughter nucleus?
A. 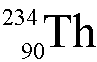
B. 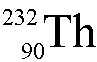
C. 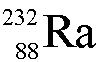
D. 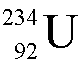
Answer
Walker5e EYU 32.1
Consider a stable nucleus with a proton number of roughly 70. Rank the numbers N, Z, and A for this nucleus in order of increasing value. Indicate ties where appropriate.
1. Z < N = A
2. A = Z < N
3. Z < N < A
4. Z < A < N
Answer
Walker5e EYU 32.2 mod
A given nucleus can undergo alpha decay, beta decay, or gamma decay. Rank the mass numbers A of the daughter isotopes for each case. Indicate ties where appropriate.
1. Aalpha < Abeta = Agamma
2. Abeta = Agamma < Aalpha
3. Agamma < Abeta < Aalpha
4. Agamma < Aalpha = Abeta
Answer
D. strontium-82
The number at the lower left is the atomic number Z and identifies
the isotope as strontium. The number at the upper left is the mass number A and it tells you the
number of nucleons is 82.
B. 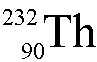
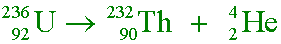
3. Z < N < A
For heavy nuclei the neutrons N outnumber the protons Z, and the mass number A is the sum of N and Z, so it is the largest of them all.
1. Aalpha < Abeta = Agamma
The alpha particle represents 4 nucleons, so alpha emission leaves behind a daughter nucleus with four fewer nucleons. Beta and gamma decays leave behind daughter nuclei with unchanged mass number A, hence Abeta = Agamma. Notice that the actual mass of the daughter nucleus mdaughter = mparent − Δm, and the value of Δm depends on both the mass of the ejected particle and the energy loss E of the nucleus, because E = mc².



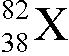 ?
?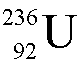 ) undergoes alpha decay.
What is the daughter nucleus?
) undergoes alpha decay.
What is the daughter nucleus?