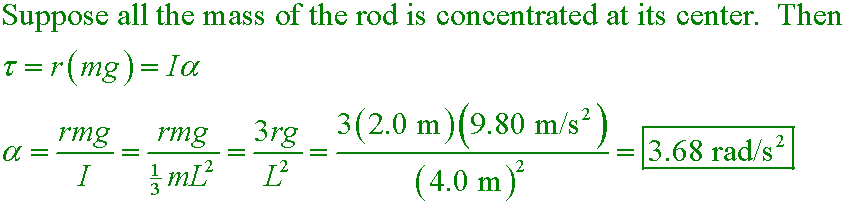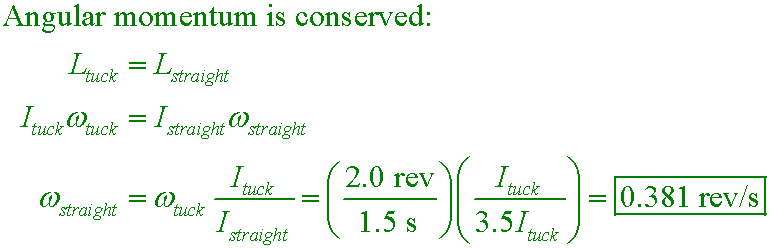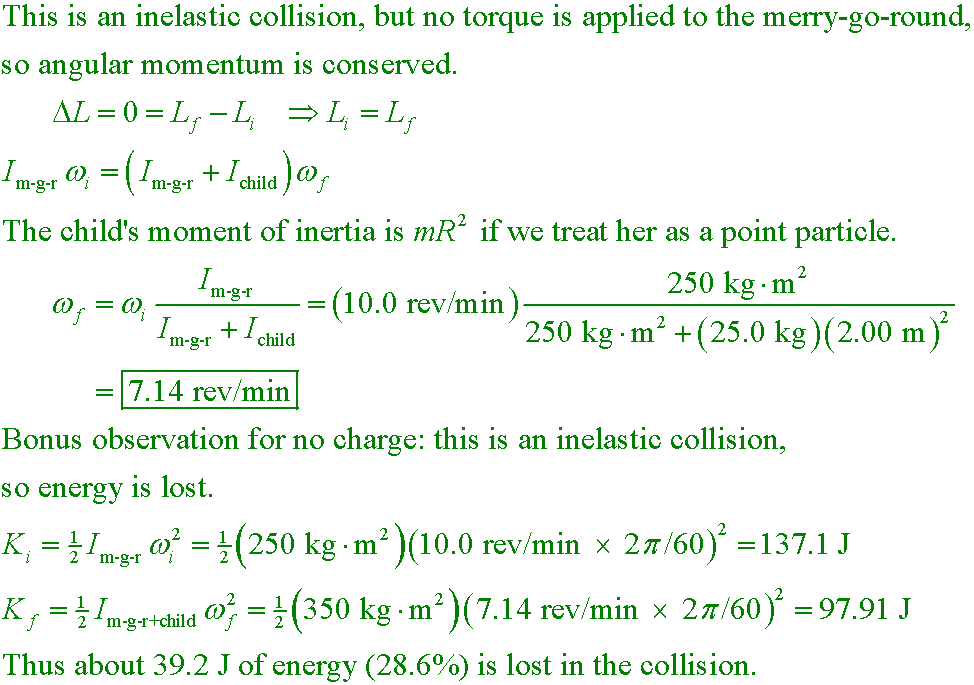Lecture 26

Summary

Newton's second law for rotation
Rolling motion
Torque & vector cross product

Bonus Question Ch. 10

Hurricane Florence over the Atlantic ocean in 2018, as photographed
by astronauts on the International Space Station. Credit: NASA

Angular momentum

definition
Newton's second law
rigid objects
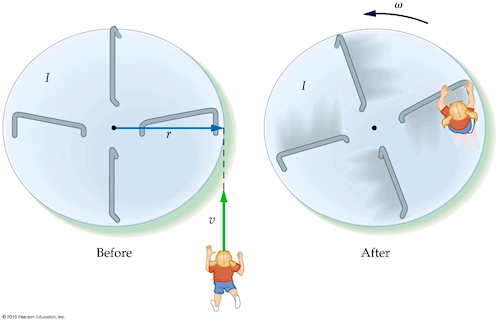
L = Iω

Example #1

Conservation of angular momentum

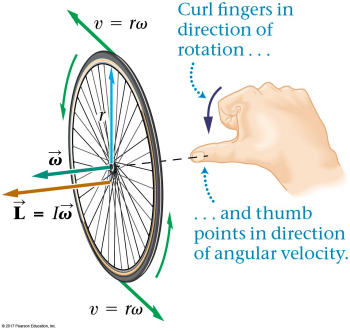
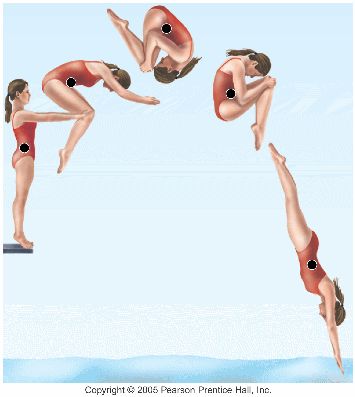
hurricanes, orbits, ice skaters, reason for the seasons
JavaLab Earth seasons applet
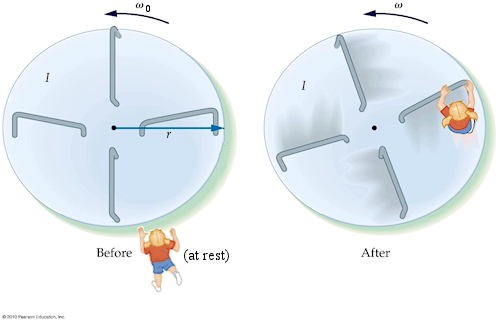
This applet is not fancy, but illustrates how the Earth's angular momentum vector points in the same direction always, leading to the seasons.
examples

demos and applet
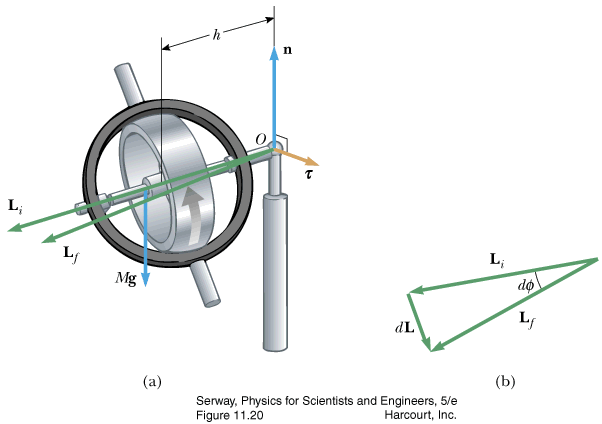
gyroscopes and precession [skipped] video

I have not yet found an HTML5 equivalent to the skater applet. Sorry!

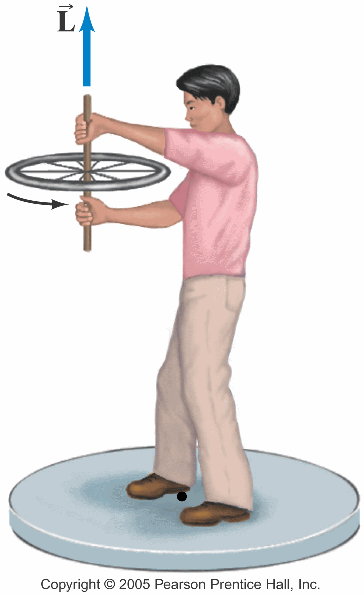
demonstration of angular momentum conservation

PoP5 Ex10.11mod
A 4.00-m-long rod is hinged at one end and initially held in the horizontal position. What is its angular acceleration upon release?
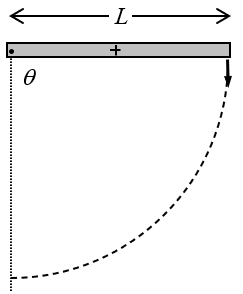hinged rod held horizontal

A. 3.68 rad/s²
B. 2.45 rad/s²
C. 6.75 rad/s²
D. 4.90 rad/s²
Answer

gc6 8.52
What is the angular momentum of a 2.8-kg uniform cylindrical grinding wheel of radius 18 cm when it rotates at 1500 rev/min?
A. 14.2 kg·m²/s
B. 7.13 kg·m²/s
C. 2.81 kg·m²/s
D. 0.233 kg·m²/s
Answer

A spinning ice skater brings her arms in closer and spins faster. As she spins faster, she gains kinetic energy. Where does the energy come from?

A. The earth's rotational energy
B. She does work to pull her arms in
C. Friction does work on her skates
D. Gravity does work on her arms
Answer

gc6 8.54
A diver can reduce her moment of inertia by a factor of about 3.5 when changing from the straight position to the tuck position. If she makes 2.0 rotations in 1.5 s when in the tuck position, what is her angular speed in the straight position?
springboard diver

A. 2.62 rev/s
B. 1.33 rev/s
C. 0.381 rev/s
D. 0.167 rev/s

Answer

Walker4e Actv.Ex.11-5
A 34.0-kg child runs with a speed of 2.80 m/s tangential to the rim of a stationary, R = 2.31 m, I = 512 kg·m² merry-go-round. What is the angular speed of the system after the child jumps on?
child runs and hops on merry-go-round

A. 0.317 rad/s
B. 0.512 rad/s
C. 1.41 rad/s
D. 3.50 rad/s
Answer

PoP5 10.46
An R = 2.00 m, I = 250 kg·m² merry-go-round rotates at 10.0 rev/min. Facing the axle, a 25.0-kg child hops on the merry-go-round and sits down on the edge. What is the new angular speed of the merry-go-round?
child sits on spinning merry-go-round

A. 5.10 rev/min
B. 8.33 rev/min
C. 14.0 rev/min
D. 7.14 rev/min
Answer

answer

A. 3.68 rad/s²

answer

B. 7.13 kg·m²/s
solution equation

answer

B. She does work to pull her arms in

answer

C. 0.381 rev/s
solution equation

answer

A. 0.317 rad/s
solution equation

answer

D. 7.14 rev/min
solution equation