Summary

• Optics
• Diffusion

---

Chapter 30

---




• Blackbody radiation applet
• experiment
• Planck's theory
• quantized energy
Example #1
Example #2


 



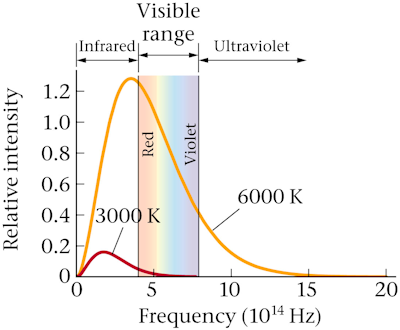
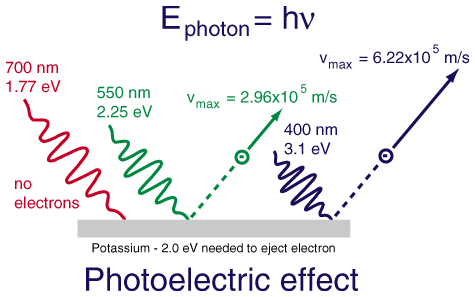
• The photoelectric effect applet

• experiment another applet
• the cutoff frequency
• KE_max and intensity
• KE_max and frequency
• instant photoelectrons
• Einstein's theory
• quantized light
Example #3
Example #4
Example #5




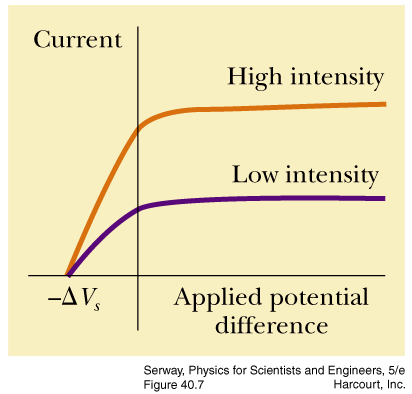
• wave-particle duality info & graphs




• Lecture learning outcomes
  A student who masters the topics in this lecture will be able to:
• describe the changes in the total energy emitted by a hot object and the frequency of the radiation it emits most strongly as the temperature of the object increases
• explain how the blackbody radiation spectrum led to the understanding that energy is quantized
• use algebra to find the frequency emitted most strongly ƒ_peak or temperature T for a blackbody when any one of these quantities is given
• describe how the results of photoelectric effect experiments led to the photon theory of light
• use algebra to find the photon energy E or frequency ƒ when any one of these quantities is given
• use algebra to find the maximum kinetic energy K_max of photoelectrons, photon energy E, or work function W₀ for a metal when any two of these quantities is given



• Practice:
  Try these additional examples
Example #6

Example #7



• Prepare:
  Read textbook section 30-5 before the next lecture

kw4 22.q.34
Stars emit light according to their temperatures. Which star would be the hottest?
A. red
B. yellow
C. white
D. blue
Answer















gc6 27.9 mod
What is the peak frequency of the EM radiation issuing from the pupil of the human eye, assuming an eye temperature of 37°C?
A. 1.82×10¹³ Hz
B. 3.34×10¹⁵ Hz
C. 5.88×10¹⁰ Hz
D. 1.90×10⁸ Hz
Answer















kw5
Light striking a clean metal produces photoelectrons. If the light is made twice as bright, what happens to the average kinetic energy of the photoelectrons?
A. The energy doubles.
B. The energy stays the same.
C. The energy is cut in half.
D. It's impossible to say.
Answer















kw5
Blue light shines on a metal, producing photoelectrons. When a red light is used the maximum KE of the photoelectrons
A. increases.
B. decreases.
C. stays the same.
D. Depends on the light intensity.
Answer















sj9 40.21
Electrons are ejected from a metallic surface with speeds ranging up to v_max = 4.60×10⁵ m/s when light with a wavelength of λ = 625 nm is used. What maximum wavelength (minimum energy) light will produce photoelectrons from this surface?
A. 756 nm
B. 897 nm
C. 962 nm
D. 1064 nm
Answer















Walker5e 30.03
The average surface temperature of the Earth is 288 K. Find the frequency at which the Earth emits radiation most strongly, and report the corresponding wavelength.
A. 735 nm
B. 1.47 µm
C. 17.7 µm
D. 204 µm
Answer















Knight2 39.stt.1
The work functions for metals A, B, and C are 3.0 eV, 4.0 eV, and 5.0 eV, respectively. The same ultraviolet photons of energy 8.0 eV shine on all three metals, creating photoelectrons. Rank in order, from largest to smallest, the maximum kinetic energy of the photoelectrons from A, B, and C.
A. KE_{max, C} > KE_{max, B} > KE_{max, A}
B. KE_{max, B} > KE_{max, A} = KE_{max, C}
C. KE_{max, A} = KE_{max, B} = KE_{max, C}
D. KE_{max, A} > KE_{max, B} > KE_{max, C}
Answer















 



D. blue
The bluer the light, the hotter the object! An object that glows blue or bluish-white is hotter than an object that glows white.




















 



A. 1.82×10¹³ Hz

[Graph of spectral power emitted by the T = 5800 K Sun in terms of frequency and wavelength:]





















 



B. The energy stays the same.
The average kinetic energy of the emitted photoelectrons depends only upon the frequency of the light. Making the light brighter produces more photoelectrons, but they have the same energy distribution as before.




















 



B. decreases.
Red light is composed of photons that have a lower frequency and thus less energy than blue photons. The emitted photoelectrons will therefore have less energy when red light is used.




















 



B. 897 nm





















 



C. 17.7 µm

This wavelength is in the infrared portion of the electromagnetic spectrum. This radiation plays a major role in the cooling of Earth's surface and atmosphere at night.
























 



D. KE_{max, A} > KE_{max, B} > KE_{max, C}
The maximum kinetic energy (which is proportional to the "stopping potential" mentioned by some textbooks) equals the difference between the photon energy and the work function. The smallest work function therefore corresponds to the largest kinetic energy of the photoelectrons.